A much simpler alternative to continuous vibration sensors (displacement or acceleration) and monitoring equipment suitable for less critical applications is a simple mechanical switch actuated by a machine’s vibration. These switches cannot, of course, quantitatively analyze machine vibrations, but they do serve as qualitative indicators of gross vibration.
The following photograph shows a Robertshaw “Vibraswitch” unit:

This switch works on the principle of a weighted lever generating a force when shaken. A pair of magnets located at the weighted end of the lever hold it in either the “reset” (normal) or “tripped” position:
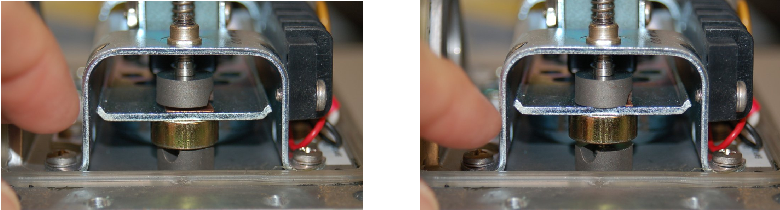
When reset, the lever is pre-loaded by spring tension to flip to the “tripped” position. All it needs to make that transition is enough acceleration to generate the “breakaway” force necessary to pull away from the holding magnet. Once the acceleration force exceeds that threshold, the lever moves toward the other magnet, which holds it securely in position so that switch will not “reset” itself with additional vibration.
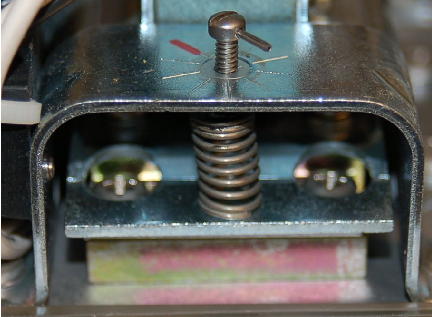
This pre-loading spring is adjustable by a small screw, making it possible to easily vary the sensitivity of the switch:
24.5 Review of fundamental principles
Shown here is a partial listing of principles applied in the subject matter of this chapter, given for the purpose of expanding the reader’s view of this chapter’s concepts and of their general inter-relationships with concepts elsewhere in the book. Your abilities as a problem-solver and as a life-long learner will be greatly enhanced by mastering the applications of these principles to a wide variety of topics, the more varied the better.
- Newton’s Second Law of motion: F = ma, describing how the acceleration of an object is directly proportional to the amount of applied (resultant) force and inversely proportional to its mass. Relevant to the calculation of force developed on a machine part from the acceleration and deceleration of vibration.
- Differentiation (calculus): where one variable is proportional to the rate-of-change of two others. Differentiation always results in a division (quotient) of units. Relevant to conversion from position to velocity, and from velocity to acceleration: v = dx dt and a = dv dt .
- Integration (calculus): where one variable is proportional to the accumulation of the product of two others. Integration always results in a multiplication of units. Relevant to conversion from acceleration to velocity, and from velocity to position: v = ∫ a dt and x = ∫ v dt.
- Fourier transforms: any repetitive waveform, no matter what its shape, is mathematically equivalent to a series of sinusoidal (sine and cosine) waves of different frequencies, amplitudes, and phase shifts added together. The frequencies of these sinusoids are all integer multiples, called harmonics. Relevant to decomposing vibrational wave signals into their constituent harmonic frequencies, to determine which parts of a machine are vibrating most.
References
Kaplan, Wilfred, Advanced Mathematics for Engineers, Addison-Wesley Publishing Company, Reading, MA, 1981.
Smith, Steven W., The Scientist and Engineer’s Guide to Digital Signal Processing, California Technical Publishing, San Diego, CA, 1997.
White, Glenn D., Introduction to Machine Vibration, version 1.76, part number 8569, DLI Engineering Corp., Bainbridge Island, WA, 1995.